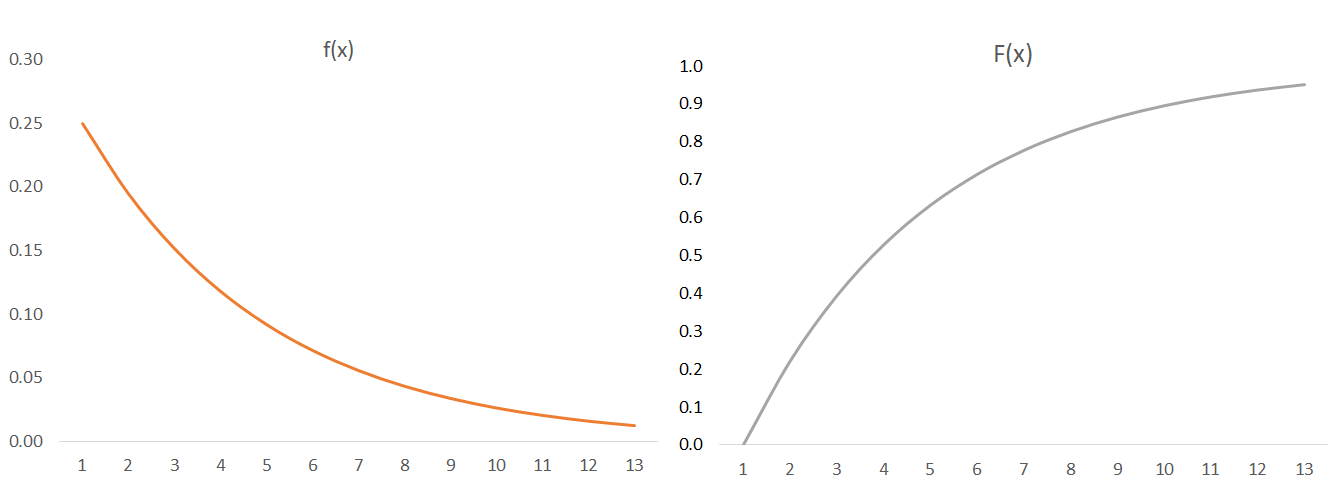
Exhibit 34.16 Exponential probability distribution, λ = 0.25.
How long will it take for a customer to arrive at a vending machine? How long will
it take for a customer to arrive at an ATM? How much time is the customer likely to spend at the ATM?
How long will a customer’s call last on a hotline?
These queries are concerned with the time for a given event to occur, or the
duration of an event. They are often modelled using the exponential distribution, Exhibit 34.16.
The exponential distribution is memoryless. The duration of an event
such as a call on a hotline, does not depend on how much time has already elapsed since the previous
call.
In queuing theory, the service time (e.g., the time it takes for a service staff to attend
to a customer) is often modelled as an exponentially distributed variable. Likewise, the arrival of
customers is modelled by the Poisson distribution, if the arrivals are independent and distributed
identically.
Note: The length of a process may comprise several independent tasks, for instance,
the number of calls made at the same time to a hotline. This follows the Erlang distribution, which
is the distribution of the sum of several independent exponentially distributed variables.
The memoryless property makes the exponential distribution appropriate for modelling the
constant hazard rate portion of the bathtub curve, used in reliability theory. For instance, the
failure of a device such as a laptop, over the time period the failure rate is constant. It
is however, not suited for modelling the lifetime of the device, because more failures occur when the
device is relatively new and when it gets old.
A random variable X is said to be an exponential random variable with rate parameter λ ( > 0) if it
has the pdf:
$$f(X)= λe^{-λx} \,for \,x>0$$
$$E(X)= \frac{1}{λ} $$
$$Var(X)= \frac{1}{λ^2} $$
$$F(x)=1-e^{-λx}$$
Note: Excel’s exponential distribution function is EXPON.DIST (x, λ, cumulative).