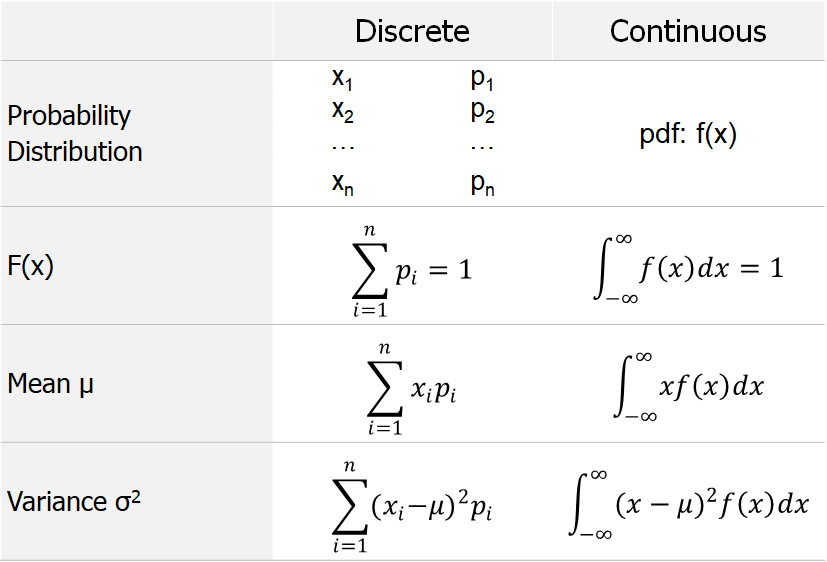
Exhibit 34.14 Comparison between discrete and continuous distributions.
A comparison between discrete and continuous probability distributions is given in
Exhibit 34.14. The probability distribution of a continuous random variable, X, is described
by its probability density function (pdf), f(x).
$$Mean \,μ = \int_{-∞}^∞ x f(x)dx$$
$$Variance \,σ = \int_{-∞}^∞ (x-μ)^2 f(x)dx$$
For a given time, t, the cumulative distribution function (cdf) F(t) of a
continuous random variable, X, is defined by:
$$F(t)=P(X≤t)=\int_{-∞}^t f(x)dx$$
$$F(-∞)=0,\,F(∞)=1 $$