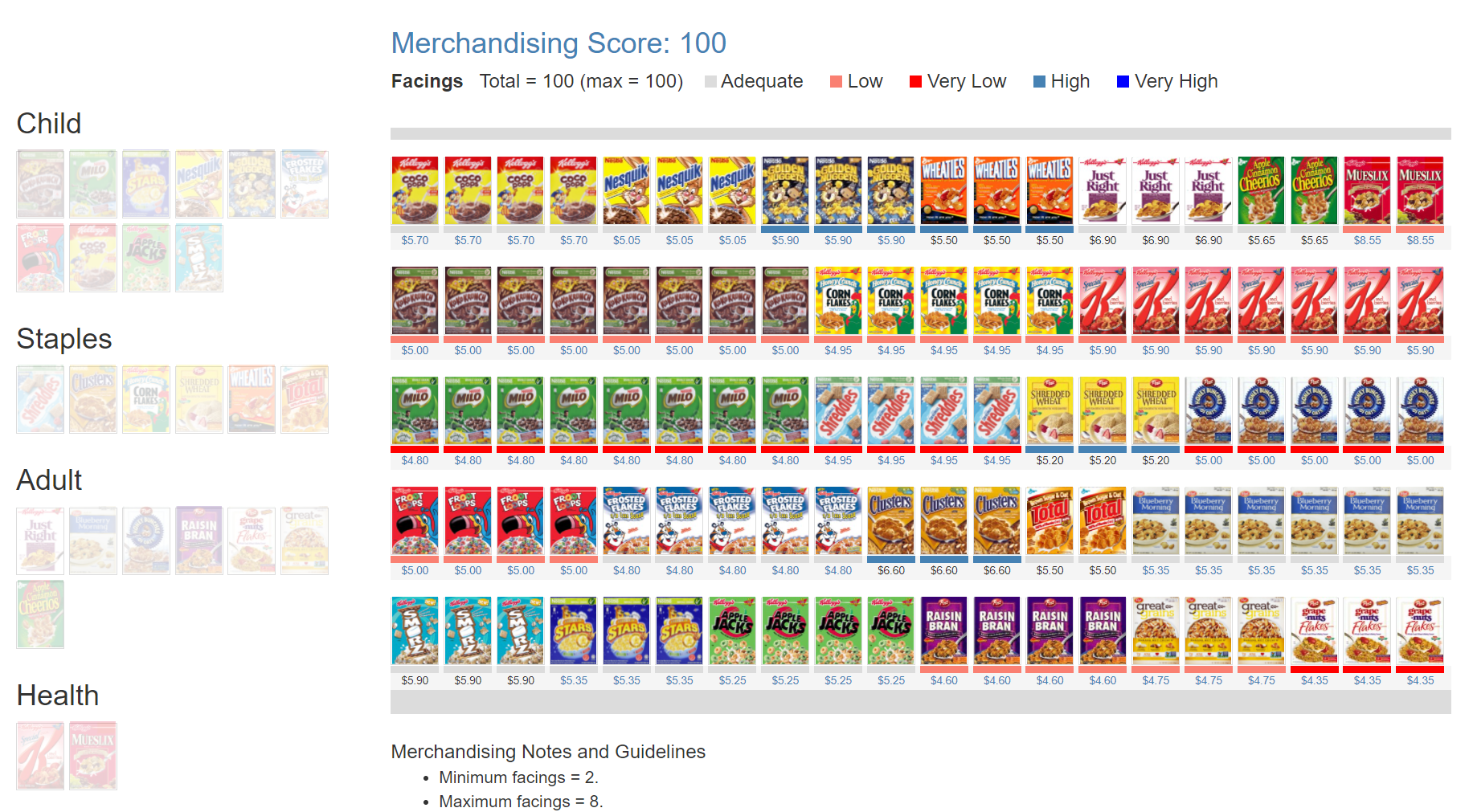
Managing Assortment — Number of Items Stocked

Brands Stocked in Store

For instance, consider a hypothetical example of flat screen TVs, which are available in 90% of stores that carry consumer durables. In this market, there are only three brands: Panasonic, Philips, and Sharp. Their presence in stores is as follows:

• Panasonic is in 60% of stores.
• Philips is in 40%.
• Sharp is in 80%.

Now, let us assume there are exactly 100 stores. This means that out of the 100 stores, 60 carry Panasonic, 40 carry Philips, and 80 carry Sharp. The cumulative distribution count for the three brands is 180 (60+40+80) across the 90 stores that carry flat screen TVs. Therefore, on average, each store carries 2 brands (180/90).

As the arithmetic demonstrates, the average number of brands stocked in stores (within a specific category) is calculated by summing up the numeric distribution of the brands and dividing it by the overall numeric distribution of the category: $$\frac{\text{Sum of Brands Distribution}}{\text{Product Category Distribution}}$$

• Sum of Brands Distribution: 60 + 40 + 80 =180
• Product Category Distribution: 90
• Average number of brands = 180/90 = 2

Items Stocked in Store

Similarly, the average number of items stocked in stores:

Brand Range Stocked in Store

And the average number of a brand’s items stocked in the stores carrying the brand:

Example: Let us consider, hypothetically, a brand that has a numeric distribution of 80% (width of distribution), meaning it is available in 80% of stores. Within those stores, the brand offers three different items, each with its own distribution: 80%, 50%, and 70%.

To compute the average number of items stocked in stores carrying the brand, we follow these steps:

1. Calculate the sum of the brand’s items distribution:
   80% + 50% + 70% = 200.
2. Determine the average number of items stocked (depth of distribution): $$\frac{\text{Sum of Brand’s Items Distribution}}{\text{Brand Distribution}}=\frac{200}{80}=2.5$$

Based on this calculation, on average, stores carrying the brand would stock approximately 2.5 of its items.